Building a two-sector equatorial platform
for a Dobsonian telescope.

Nederlandse versie (Dutch version)

To calculate the dimensions, you can download a spreadsheet (Excel) or a program in TurboPascal.
The spreadsheet will give you some more degrees of freedom in constructing the platform. On page 2 and 3 of the spreadsheet are calculations for platforms with one pivot point at the South and one sector (North).

Why an equatorial platform?

The most important disadvantage of a dobsonian mount is that the telescope doesn't follow the stars, or, more exactly stated: a dobsonian mount does not compensate for the rotation of our planet. This disadvantage is especially noticable at high magnifications. A planet or other celestial object will run out of the field of view in a few seconds, which makes detailed observation very difficult, if not impossible. The solution is a so called equatorial platform: a kind of low table of which the top, driven by a small stepper motor, compensates for the movement of earth. The most common equatorial platform has a circle sector at the North side and a fixed pivot at the South side. The mount discussed in this article has two circle sectors: one North and one South. This leads to more stability especially with large telescopes, with a relatively high centre of gravity. Another advantage is, that this type of mount is more compact and therefore easier transportable. More information about this type of equatorial platform (or cylindrical bearing platform) is to be found on Chuck Shaw's website. The difference between Chuck's article and this article is, that this article contains more detailed information about constructing the most difficult components of the platform. Another difference is the type of drive: Chuck Shaw describes a tangent-arm drive, in this article a direct roller drive is described. Information about the one-sector type is to be found on the website of Robert Duvall (see links page for URL's).

An equatorial platform is meant for visual observing in the first place. If well constructed and perfectly polar aligned unfollowed short exposure photography and piggy-back photography is possible as well.

Two platforms

The platform described here was built for my 12 inch Dobsonian. For my 20 incher I built one slightly different. Most important differences: only one south bearing (instead of four) and a tangent arm drive instead of a roller drive. Pictures of this plaform and short explaining notes are here

Working principle.

As everybody knows, Earth rotates around its axis in 24 hours or 15 degrees per hour. An equatorial mount compensates for this movement by revolving the telescope around the same axis, with the same speed in the opposite direction. This is easy to observe in a common equatorial mount: the telescope actually hangs on this axis. In the case of an equatorial platform the telescope does not hang on the axis, but stands on a low table below the axis. The top of the table performs part of this movement: in most cases about 15 degrees or, expressed in time: about one hour. This is shown in picture 1, below.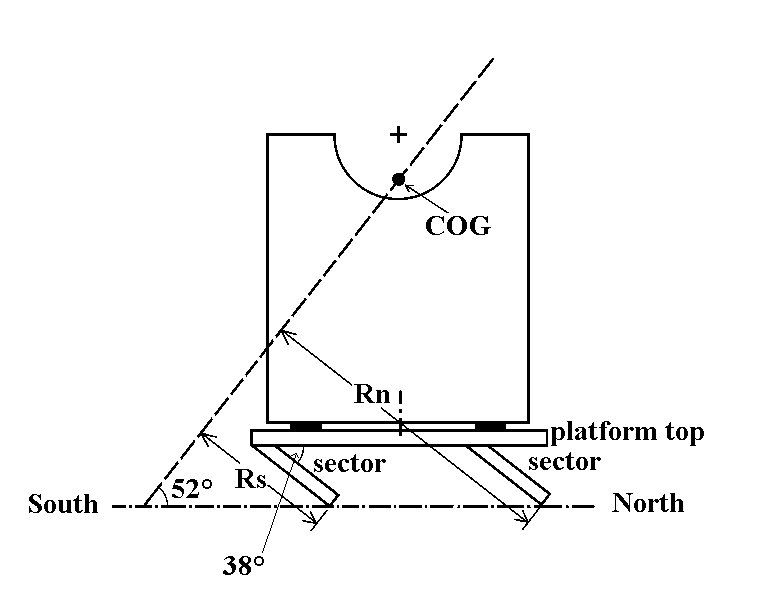

figure 1.

From the circles (of which Rn and Rs in figure1 are the radii of the North and South sector respectively) only the sectors under the 'table' top (which we will call platform top from now on), are used. If the platform top rotates too far, the telescope will fall from the table. At the start of an observing session the platform top is set to its most westerly position. After about one hour of observing, when the platform top has reached its most easterly position, it has to be reset to the starting position.

As also shown in figure1, the virtual polar axis passes through the center of gravity (COG) of the telescope and mount assembly. In most cases the COG will be a small distance below the pivot center of the altitude bearings. This is not very critical, but if the virtual polar axis passes through a point too far above the center of gravity the motor will have to push the telescope uphill and it is not unlikely that the movement will stop in that case. If the virtual polar axis passes through a point too far below the center of gravity, the telescope will be top-heavy and, in the worst case, will fall from the platform in its most extreme (East or West) position.

components of the equatorial platform

An equatorial platform consists of the following components:

The dimensions of the components are depending on:

Before making the circle sectors it is very important to calculate the radii of the circles from which they have to be cut. To calculate the radius we depart from the geographical lattitude of the place where the mount will be used and from the known dimensions of the telescope-dobsonian mount combination: lenght and width of the dobsonian mount and heigth of the center of gravity of this combination. By means of a large number of goniometric calculations the dimensions of the sectors can be calculated. This is quite a lot of work and if you want to change something on second thought, everything has to be calculated again. To simplify this job I wrote a computerprogram (in Turbo Pascal) and, for ATM prefering a spreadsheet, this spreadsheet will do the job. After entering the dimensions you already know, the radii of the circles and the dimensions of the sectors are calculated. The relevant input variables for this platform are shown in figure 8. The program also askes whether you want to enter steppermotor specifications (to calculate the reduction rate). This is only relevant for the tangent-arm driven platform as described by Chuck Shaw and not for the roller drive described in this article. The same is true for a third sector the program calculates: the drive-arm. It's best to enter 0 (zero) for this parameter and to ignore the information about this drive-arm and the reduction figures in the output.

In this article the construction of every component will be treated in detail. It will not be necessary to use advanced tools. If you have advanced tools at your disposal the construction of some of the parts will be a little easier. The most advanced tool I used were an electric drill in a drill press and a jigsaw. The result of my building efforts is shown in this fotograph.

Materials

To illustrate the building of this type of equatorial platform I will use my own 12 inch f/6 dobsonian as an example. So, the sizes of the materials described below cannot be used for another telescope. Using the above mentioned computer program and entering the relevant dimensions of your own dobsonian, the exact dimensions for building your own platform have to be calculated. Originally the measures were in the Dutch language. To obtain inches the number of millimeters was divided by 25.41. The height of the centre of gravity of my dobsonian is 708 mm (27.9 inch). Length and width of the dobsonian mount are 520 mm (20.5 inch). My residence is situated at 52.08 degrees Northern lattitude.
Wooden materials:
Non wooden materials:

Building the platform

In this section the construction of the components will be described. As mentioned above I will use my own 12 inch f/6 dobsonian as an example.
The circle sectors and the platform top
The most difficult parts to construct (because of the fact that it has to be done very accurately) are the circle sectors, the supporting triangles and the triangle blocks on which the bearings have to be mounted. Therefore we will take our time to describe the contruction of these parts. We will start with the circle sectors .

The computerprogram/spreadsheet has calculated that the North sector and South sector (see figure 1) of my platform have to be cut off circles with radii of 26.45 inch and 12.28 inch respectively. The aluminium strips which will be mounted on the edges of the sectors are 2 mm thick. This has to be subtracted from the radii. So the resultant radii will be 26.36 and 12.19 inch respectively. The sectors are cut out of the 27.5x20.5 inch piece of plywood as follows:

At about .8 inch distance from one of the 20.5 inch sides, exactly in the middle between the two 27.5 inch sides, a point is marked: the center of an imaginary circle with a 26.36 inch radius. Then take a thin slat of of 27.5 inch length and hammer a small nail in it, close to one of the ends. This will be the pivot of a hand-made compass. At exactly 26.36 inch distance from the pivot a small hole is drilled with the same width as the point of a pencil. Push the point (nail) of the compass in the marking en draw the arc of the first (Northern) circle on the piece of plywood. After that the center of the other circle is marked at about 13 inch distance from the side close to which you drew the first arc. Adjust the compass to a length of 12.19 inch and draw the arc of the Southern circle sector at the opposite side of the piece of plywood. With a jigsaw both arcs are cut out of the plywood. Take care to stay one or two millimeters on the outside of the lines. The result is shown in figure 2A. Now for the finetuning of the arcs: the arcs have to be made smooth with a router, exactly on the lines drawn with the compass. Therefore a router is placed in the drill. Opposite to the drill a small wooden platform is build, such that the plywood with the roughly cut sectors can be placed upon it with one of the arcs against the router. (see figure 2 B t/m F). It is very important that the center of the circle (where the pivot was while drawing), the exact center of the plywood and the center of the router are exactly aligned. (see figure 2A). Only then the router will exactly follow the line drawn with the compass. The distance of the pivot of the plywood to the router is exactly 26.36 and 12.19 inch for the North and South sector respectively. Fasten the plywood with a nail to the wooden platform when in the exact position. Turn the plywood to one side, lower the router into the right position, start the drill and slowly move the plywood to the other side. The plywood has to be moved in the direction opposite to the rotation direction of the drill. So if the drill rotates clockwise, the plywood has te be moved anti-clockwise. When ready, the arcs will be perfectly smooth. The next step is cutting the arcs from the piece of plywood. This has to be done very carefully, because at equal distances from the center of the arc, the distance form the arc-side to the straight side of the sector have to be exactly the same or, stated otherwise: the tapering from the midpoint to both sides has to be the same. The program calculates the width of the arcs: in this case 3.2 inch at the center. The length of the North arc is the same as the width of the piece of plywood (which is also the width of the platform top). The lenght of the South sector has to be 16.56 inch. Relative to this sector the platform top will protrude about 2 inch on both sides.

The arcs are smooth enough now to attach them to the aluminium strips. To attach the strips to the arcs a good two-component glue or contact glue is used. After applying the glue the strip is attached to one side of the arc with a small screw. After that it's easy to bring the strip in the correct position and fasten it with a second small screw. Of course small holes for these screws have to be drilled in advance. The balls of the ball transfer's rotate against the back side of the wooden sectors. Wood is soft and the bearings will damage the wood. Therefore it is necessary to cover these sides with some kind of hard plastic ar metal. Do not use aluminium, this is too soft.

The sectors are ready now to be attached to the bottom of the platform top. To support the sectors six wooden triangles are cut out of the remains of the .47 inch plywood, with a sharp angle of 38 degrees (for 52 degrees lattitude). The triangles are attached to the sectors with a good type of glue; one in the middle and one on each side of this, halfway between the center and the end of the sector. The last step is attaching the sectors and triangles to the bottom of the platform top. The distance between the sectors has to be exactly as planned: in this example the inner rim of the North sector has to be at a distance of exactly 20.5 inch from the outer rim of the South sector, as measured alongside the bottom of the platform top. (see figure 2, G). What the platform top looks like after mounting the sectors is shown in this photograph.

The bearings and the drive roller
Now that the platform top is ready the blocks on which the bearings will be mounted have to be made. Those triangle blocks are about 1.6 inch wide with angles of 90, 52 and 38 degrees. The 38 degree angle has to be exactly 38 degrees (the exactness of the other two angels matters less), because this angle has to be exactly the same as the angle between the sectors and the bottom of the platform top. If not, the rollerblade bearings will only touch the aluminium strips with one edge and not with their whole width. The height ot the blocks has to be about 2 inch with the 90 degree angle at the top. It's very important to check for the exact angle of this blocks. To do this put the platvorm on a table or on the floor, resting on the two sectors and position the blocks under the platform, against the backside of the sectors, where they have to fit perfectly. Mistakes can be corrected by sandpapering or filing the blocks to the right form. When the blocks are ready the bearings (two for each block) have to be mounted. There are eight bearings:
  1. Four 'follow' bearings which roll against the aluminium strips. For this purpose the rollerblade bearings are used. One of these bearings, the North-West one, will be converted to a rollerdrive.
  2. Four 'support' bearings, rolling against the metal or plastic-covered backside of the sectors. For this purpose the ball transfer's are used.

Instead of ball transfer's rollerblade bearings can be used as support-bearings as well.(see figure 6). This will make the construction a bit more complicated however: de 90 degree angle of the bearing mounting-blocks has to be exactly 90 degrees in that case. To prevent slippage the bearings will have to be canted inwards at the same angle as the tapering of the sectors at the point of support, while their rotation axis points to the polar axis.(see figure 6C). If you choose to use this option you will have to purchase bearings with sufficient outside diameter to prevent the axis from having to be mounted to close to the rim of the block.

To mount the bearings, holes will have to be drilled in the sides of the blocks that will be pointing to the sectors after assembling. The holes have to be drilled exactly at an angle of 90 degrees with respect to the surface of the block for the bearings to be able to work properly. It is very difficult to do this with a handheld drill. Therefore we use the small pieces of wood (1.6x3 inch) from the wooden materials list, which can be positioned under a drill in a drillpress, which makes the job easy. The result is shown in this fotograph.

The holes for the ball transfer's (support bearings) are drilled first. The center of the holes are at 1 inch distance from one of the 1.6 inch sides of the pieces of plywood, at equal distances from the two 3 inch sides. After that, in three of the four pieces of plywood .3 inch holes are drilled to mount the shafts (made of .3 inch threaded rod) of the follow-bearings (rollerblade bearings). Depending on the width of the ball transfer's, the distance from the center of the hole of the ball transfer and the threaded rod hole may vary. The diameter of the holes for the ball transfer's I used were .9 inch, the distance between the center of these holes and the threaded rod holes .75 inch. The holes for the ball transfer's are above the threaded rod holes. The lower hole in the fourth piece of plywood is .25 inch instead of .3 inch. In this hole the driveroller shaft will be mounted. The distance form the midpoint of the .25 inch hole to the center of the hole of the ball transfer, depends on the diameter of the drive-roller to be used. The roller I used had a .55 inch radius, .12 inch more then the radius of the rollerblade bearings. Therefore the center of the hole for the driveroller shaft is .12 inch lower with respect to the center of the hole of the ball transfer. The three pieces of plywood are glued to the triangle support-blocks. The piece of plywood with the rollerdrive is not glued but screwed to the block, because it will be mounted adjustable later to be able to manipulate the pressure of the roller against the aluminium strip to prevent slippage. It's important to mount the pieces of plywood in such a way that, when placed next to each other, the centers of all four ball transfer's are at the same height. The upper point of the three rollerblade bearings and the drive roller have to be at exactly the same height as well.

The ball transfer's are placed in the .9 inch holes (they may be glued but this is not necessary). In the .3 inch holes pieces of threaded rod (of about 1.6 inch length) are glued. The rollerskate bearings are mounted on these shafts. To compensate for the height of the ball transfer's a nut and maybe some washers have to be placed on the threaded rod under the rollerblade bearing (see this photograph).

The drive-shaft
. The diameter of the drive shaft (of my platform) is .2 inch. The thickness of this shaft depends on the diameter of the central hole of the wormwheel and the diameter of the central hole of the driveroller. To prevent slippage the roller has to be attached to the shaft very solidly. To achieve this I drilled a .12 inch hole through the shaft in which a .6 inch long iron bar was glued. This bar was fitted in the lower side of the rubber roller by cutting some rubber material off with a sharp knife. Two component glue was used to glue the roller to the shaft. The two roller bearings to suport the shaft are mounted on the the outside of the plywood, one on each side of the shaft-hole, with two-component glue. To do their work smoothly the bearings have to be positioned exactly opposite to each other. The wormwheel is mounted on the other end of the shaft. The lenght of the rollerdrive assembly has to be such that the platform top in its most easterly position does not touch the wormwheel.
The ground board
Now that the platform top, the bearing assemblies and the the drive-roller assembly are ready the four assemblies can be positioned on the ground board. The blocks on the North side must be a bit further apart then the blocks on the South, because the North sector is a bit wider and less tapered. The blocks are placed roughly in position on the groundboard and the platform top is placed upon the bearings, which may, if necessary, be supported by some heavy tools or stones to prevent them from slipping away. After that you will have to play a little with the exact location of the assemblies to get them in the right position: take your time. While doing this it's important to take into account that: When in correct position the four assemblies can be glued to the ground board. Figure 5A (not drawn to scale) shows the ground board with the bearing assemblies and the drive-roller assembly.

The plywood plank with the driveroller and wormwheel assembly were screwed (and not glued) to the triangle block to be able to be able to make this assembly adjustable. Proceed as follows:

Sometimes slippage will accur nevertheless, for instance when the aluminium strips become wet in a moisty atmosphere and when rotating the telescope in azimuth. If so, take one or more of the following actions:

  1. Check whether the center of gravity of the telescope-dobsonian mount combination is exactly above the center of the platform top (the rotation center). If not, the telescope has to be pushed uphill, which can cause slippage (or even blocking of the movement).
  2. Reduce the friction of the azimuth rotation by swapping two pieces of teflon for ball transfer's (see dobsonian tips). Friction will be reduced to about one-third of the original friction.
  3. Glue a strip of fine abbrasive (for instance a part of a power-file belt) on the part of the aluminium strip in contact with the roller.
The drive motor.
To drive the platform a 12 Volt steppermotor will be used, preferably one that makes 200 steps per revolution (is 1.8 degrees per step). You will need a stepper motor with five or six wires. Stepper motors making less steps per revolution can be used as well, but they have to run at higher speed to prevent the bigger steps to show up in the eyepiece as vibrations. A speed of 20-25 revolutions per minute (65-85 steps per second) will do for a 1.8 degrees steppermotor. For a 7.5 degrees stepper 80-100 rovolutions per minute will be necessary.

To control a steppermotor an electronic circuit is needed. I used the circuit as published by Robert Duvall. This circuit is easy to build and easy to control. Another advantage of this circuit is, that it's easy to calculate the speed of the stepper motor, given the values of the risistors and capacitors used. When the specifications mentioned in the above mentioned article are used the speed is way too low. An important modification of the circuit is to change part of one of the resistors (Rb in the circuit) for a pot to be able to finetune the speed. In figure 3 the x-axis shows the number of steps per seconds of the stepper motor. The y-axis shows the calculated value of the resistor Rb for each numer of steps. To achieve a speed of 25 revolutions per minute the value of the resitor Rb lies between 14000 and 15000 ohm. Using a 14000 ohm resistor and a 1000 ohm pot it is possible to optimally control the speed. To prevent damaging the circuit I recommend to mount a diode in the beginning of the circuit. Problem with steppermotors can be, that the steps are visible in the eyepiece and the stars become stripes. This is not what we want. There's one circuit I know of, preventing this by 'smoothing' the step progression. It's designed by Nils Olof Carlin and can be found here. It's a very fine circuit, but not easy to make form someone with little soldering experience.

After building the circuit it will have to be connected to the stepper motor. One of the five (or two of the six) wires are connected to the 12 Volts electricity source or battery. The remaining four wires are control wires. It will take some trial and error to connect the wires in such a way that the stepper rotates smoothly in the right direction.

The reduction assembly.
In one hour 6.93 inch (exactly) of aluminium strip has to be pushed away by the roller to attain a accurate compensation of the rotation of earth. A 1.1 inch diameter roller (which I used) has a circumference of 1.1*pi = 3.46 inch. This means that the number of revolutions of the roller has to be no more then 6.93/3.46 = 2.005. The number of revolutions per hour of the stepper motor is 25x60 = 1500. So the necessary reduction has to be: 1500/2.005 = 748. This will be attained by using some coghwheel combinations and a worm-wormwheel combination. A cogwheel with 20 teeth is mounted on the stepper motor shaft and connected to a 50 teeth cogwheel (reduction: 2.5). Then a 10 teeth cogwheel in contact with a 20 teeth cogwheel (total reduction: 2x2.5 = 5). Next step is a reduction using cogwheels with number of teeth ratio 1:5, which brings the total reduction to 5x5 = 25. Last step in the reduction is the worm-wormwheel combination. The reduction of a worm-wormwheel combination is equal to the number of teeth of the wormwheel. The wormwheel I used has 30 teeth, which brings the total reduction to 30x25 = 750. Finetuning of the speed is possible with the potmeter.

The reduction assembly will not be mounted directly to the ground board, but on a separate piece of wood of (about) 3.5x4.7 inch. The thickness of the wood depends on the height of the wormwheel above the ground board. I had to use a .5 inch thick piece of plywood to give the worm a good grip on the wormwheel. The shafts rotate in the 90 degree iron angles (materials list), which are supported by wooden blocks. The copper bearings for the cogwheel shafts are mounted (with two component glue) in the widened or newly drilled holes in the iron angles. The cogwheels must be attach sturdely to the shafts to prevent slippage. The steppermotor is connected to one of the iron angles as well, at the beginning of the assembly. The worm is positioned at the end. Take care that the platform top does not tough one of the cogwheels or the wormwheel when positioned in most easterly position. To be able to fasten the reduction assembly on the groundboard, put the assembly in the exact position and drill a .25 inch hole through the assembly board and in the ground board. Glue a piece of .25 threaded rod in the hole in the ground board. The reduction assembly can be fastend with a .25 inch nut or wingnut and will be removable. This makes it easy to disconnect the worm and the wormwheel when, after one hour of observing, the platform has to be reset to it's starting (most westerly) position. This photograph shows the design of my reduction assembly and how the reduction assembly is connected to the stepper motor and to the wormwheel.

The finishing touch
. Exactly at the center of the platform top a hole is drilled (the width of which depends on the hole in the dobsonian mount) for the rotation shaft of the donsonian mount. On the top of the platform 3 pieces of teflon are mounted to function as azimuth bearings. To reduce friction one or two of these pads can be swapped for ball transfer's (see dobsonian tips).

Last element to be finished is the ground board. The ground board has to be ajustable east-west and north-south to compensate for irregularities of the underground and for deviations in lattitude, which is possible up to about 10 degrees. For this purpose three .3 inch nuts are glued into holes drilled in three optimally choosen positions in the ground board. With pieces of threaded rod with a wingnut glued on top it will be easy to adjust the position of the mount.

Using the platform.

The platform is ready to use now. If all activities are performed fairly accurate and the speed of the stepper motor is finetuned, the platform will follow the stars very well, if polar aligment is in order. For visual observing polar alignment is very forgiving. For optimal polar alignment I recommend using the wellknown stardrift method. Proceed als follows:
  1. Position the platform roughly north-south, using Polaris as a reference. Assuming the platform is used at the lattitude it was made for, the platform top has to be level when positioned in the middle position.
  2. Put a crosswire eyepiece in the focusser of the telescope and aim the telescope at a star as close as possible to the point where heaven-equator and meridian meet. The cross in the eyepiece has to be positioned horizontal-vertical.
  3. Movement of the star in horizontal direction is caused bij a non-optimal motor speed, but we will neglect this now (assuming that the star stays in the field of view). When the star travels up, the telescope points to far to the east. The northern side of the mount has to be replaced a bit in western direction. When the star travels downward, the opposite is true. Proceed untill there is no more travel up or down.
  4. Point the telescope at a star on the equator in the eastern sky, as close as possible to the horizon. If the star travels up, the virtual polar axis points to low and the soutern side of the platform has to be lowered. If the star travels down, the opposite is true. Again, proceed untill there is no more travel up or down.

Concluding remarks.

Building an equatorial platform is not very difficult and observing with a dobsonian is much more rewarding using such a mount. I hope this article will be helpfull to many observers. I would appreciate ATM-ers to send me an email when going to build an equatorial platform using the information provided in this article and I'll be glad to answer questions if necessary.

Like I said at the beginning of this article I also built a platform for my 20 inch telescope. Pictures of this platform and short explaining notes can be reached by clicking at the link below.

Pictures of the platform for my 20" scope